Truth, Geometry, Algebra
1. According to H.S.M. Coxeter and Richard J. Trudeau"There is a pleasantly discursive treatment of Pontius Pilate's unanswered question 'What is truth?'."
– Coxeter, 1987, introduction to Trudeau's The Non-Euclidean Revolution
1.1 Trudeau's Diamond
Theory of Truth
1.2 Trudeau's Story
Theory of Truth
2. According to Alexandre Borovik and Steven H. Cullinane
2.1 Coxeter Theory according to Borovik
2.1.1 The Geometry–
Mirror
Systems in Coxeter
Theory
2.1.2 The Algebra–
Coxeter
Languages in Coxeter
Theory
2.2 Diamond Theory according to Cullinane
2.2.1 The Geometry–
Examples: Eightfold
Cube
and Solomon's
Cube
2.2.2 The Algebra–
Examples: Cullinane
and
(rather indirectly related) Gerhard
Grams
Summary of the story thus far:
Diamond theory and Coxeter theory are to some extent analogous-- both deal with reflection groups and both have a visual (i.e., geometric) side and a verbal (i.e., algebraic) side. Coxeter theory is of course highly developed on both sides. Diamond theory is, on the geometric side, currently restricted to examples in at most three Euclidean (and six binary) dimensions. On the algebraic side, it is woefully underdeveloped. For material related to the algebraic side, search the Web for generators+relations+"characteristic two" (or "2") and for generators+relations+"GF(2)". (This last search is the source of the Grams reference in 2.2.2 above.)Update of Feb. 21, 2010--
Reflections
From the Wikipedia article "Reflection Group" that I created on Aug. 10, 2005– as revised on Nov. 25, 2009–
|
Historically, (Coxeter 1934) proved that every reflection group [Euclidean, by the current Wikipedia definition] is a Coxeter group (i.e., has a presentation where all relations are of the form ri2 or (rirj)k), and indeed this paper introduced the notion of a Coxeter group, while (Coxeter 1935) proved that every finite Coxeter group had a representation as a reflection group [again, Euclidean], and classified finite Coxeter groups. Finite fields
When working over finite fields, one defines a "reflection" as a map that fixes a hyperplane (otherwise for example there would be no reflections in characteristic 2, as −1=1 so reflections are the identity). Geometrically, this amounts to including shears in a hyperplane. Reflection groups over finite fields of characteristic not 2 were classified in (Zalesskiĭ & Serežkin 1981). |
Related material:
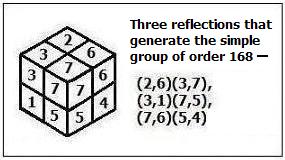
This note, "A Simple Reflection Group of Order 168," and
by Ascher Wagner, U. of Birmingham, received 27 July 1977
| Journal | Geometriae Dedicata |
| Publisher | Springer Netherlands |
| Issue | Volume 9, Number 2 / June, 1980 |
[A
primitive
permuation group preserves
no nontrivial partition of the set it acts upon.]
Clearly the eightfold cube is a counterexample.
Update of Feb. 24, 2010--
Transvections
A topic related to the above material on reflection groups over GF(2)--
Transvection groups over GF(2). See, for instance...- Binary
Coordinate
Systems, by Steven H. Cullinane, 1984
- Classification
of
the
Finite
N-Generator
Transvection
Groups
Over
Z2, by
Jizhu Nan and Jing Zhao, 2009, Advances
in
Applied
Mathematics Vol. 44 Issue 3 (March 2010), 185–202
- Anne Shepler, video of a talk on Nov. 4, 2004, "Reflection Groups and Modular Invariant Theory"
Coxeter vs. Fano
Excerpts from Coxeter's Projective Geometry sketch his attitude toward geometry in characteristic two.